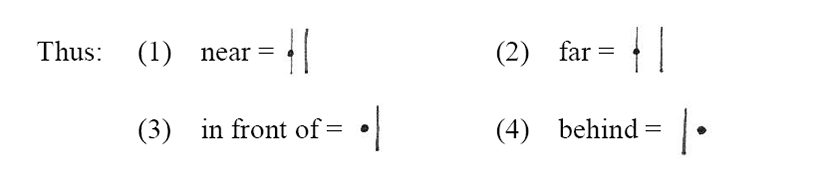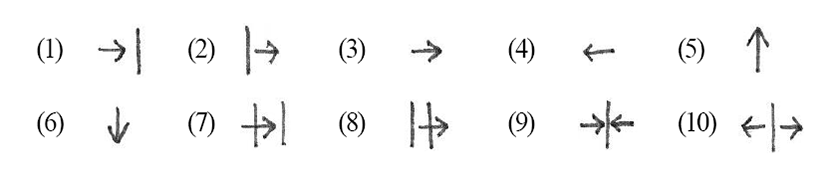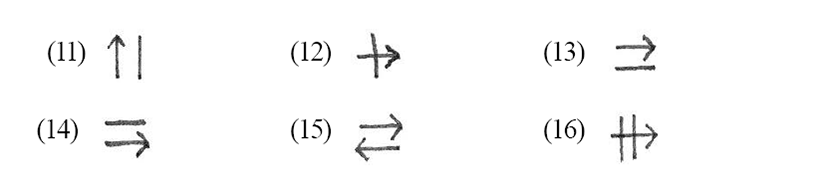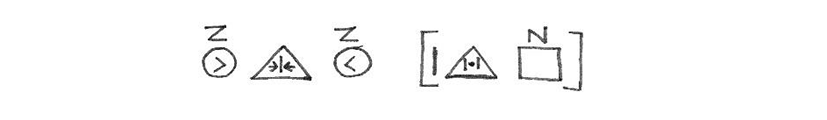1. Karl Haag: Life
Although almost entirely unknown or forgotten today, the German dialectologist Karl Haag (1860-1946) was, in his time, an eminent contributor to the field of area linguistics, as well as writing papers on the general structure of language. Born in Schwenningen am Neckar (modern-day Villingen-Schwenningen), Haag was a native speaker of the local Allemanic-Franconian dialect, and it was this that gave him an interest in dialects and language in general. In his youth he studied a number of foreign languages and could read Latin, English, French, Italian, Spanish, Dutch, and Swedish. As a teacher in a Gymnasium [grammar school] he was able to use his summer holidays to go walking and research the dialects of Germany. He also travelled in Turkey and North Africa. With such a wide variety of foreign languages at his finger-tips, he was able to turn his mind to a theory of the general structure of language and to ways of representing its logical structure. He wrote continually between 1888 and 1946 on dialectology and linguistic theory. He died in 1946 at the age of 86 in the town of Künzelsau in Baden-Württemburg, southern Germany (Benzing 1951).
Towards a Logically-based Graphic Language [1902]
Towards a Logically-based Graphic Language [Versuch einer graphischen Sprache auf logischer Grundlage, hereafter referred to as Versuch] appeared in 1902, surprisingly early in the career of a linguist, given that its aim is no less than an attempt to reveal the very basis that underlies not just the structure of German, but the structure of language in general. Published by Kohlhammer in Stuttgart, it is a slim booklet of 83 pages, written in a dense, very technical, and very individualistic style. Kohlhammer have no record today of the print-run but believe it to have been very small (Personal communication, Christina Jabs, Kohlhammer Verlag, June 2016). On the cover, his name appears as ‘Carl Haag’ but the usual spelling of his first name is ‘Karl’.
Haag had developed a symbolic language to demonstrate his theories, but the practicalities of printing meant that it was impossible to incorporate his hand-drawn symbolic examples into the text, and they had to be appended in a separate section at the end. This certainly causes difficulties for the reader, not only because he or she has to turn constantly from text to appendix while reading, but also because there is no exact match between the examples given in the text and their position in the appendix.
The book is long out of print and together with other works of Haag must be sought in libraries, on the Internet or on the premises of antiquarian booksellers. It has never been translated into English. Moreover, it does not figure in the large-scale reviews of universal and artificial languages such as Couturat & Leau (1903), Pei (1951), Large (1985), Yaguello (1991), or Okrent (2009). It is perhaps for these reasons that the work has been all but forgotten, although, paradoxically, it has an important place in the history of artificial and universal languages, as I shall attempt to demonstrate in the following pages.
Even though it is contained in a mere 70 pages of text and 13 pages of symbols and diagrams, Haag’s system is immensely complex. Any explanation of it must proceed by degrees, as each of Haag’s approaches to aspects of language is closely tied to each of the others, and a careful unravelling must be undertaken if the reader is ultimately to be able to see how the system interlocks and functions. I must therefore crave a degree of patience and indulgence on the part of that reader as I attempt to recreate Haag’s system in an intelligible and practical format. Thereafter follows a critique of the work, and links are made to contemporary work in linguistics and to work on a modern ‘Real Character’ (see Maun 2013, 2015).
3. Historical Context
Haag’s work took place at the confluence of two streams of historical and contemporary influence.
The first was that of ‘universal language’. The history of artificial languages and Real Character has been briefly outlined in two previous papers in this Journal (Maun 2013, 2015). Since the seventeenth century, there have been many attempts to find a way of representing language such that anyone, whatever his or her native language, can understand a message passed between two people. Leibniz hoped to produce such a system and referred constantly to it in his writings (Eco 1985, Davis 2000, Maat 2004), going so far as to believe that an ‘algebra of thought’ was possible, a representation of language that would enable people to discover the truth or falsehood of a statement merely by calculating, using symbolic figures. Needless to say, his efforts ended in failure. Other systems such as those of Cave Beck (1657), a schoolmaster, used numbers to represent words, set against an alphabetic list in English. The need to understand English before one could use this allegedly ‘universal’ system seems to have eluded the author.
The two great ‘universal’ systems of the seventeenth century were those of George Dalgarno (1661) and Bishop John Wilkins (1668). Each attempted to divide the world into a number of classes and thereafter represent the members of those classes by symbols. Verses containing the vocabulary, or long complicated tables acted as route-maps to enable readers to find the words that they sought. The system of symbols devised by Wilkins (‘the Real Character’) also had a spoken version (‘the Philosophical Language’) in which letters combined to form pronounceable words.
In the eighteenth century, de Maimieux’s Pasigraphie [i.e., pasigraphy, a language meant only to be read] (1797) resembled in some aspects the table-based system of Wilkins. It used a system of twelve combinable symbols and the length of a ‘word’ formed from their combination would in part define to what category of reality the word referred, while a set of tables served as points of reference. The system enjoyed some success for a while.
Such schemes, however, were destined to be eclipsed by ‘the artificial language movement’. In essence, the artificial languages produced in the 19th and 20th centuries either used grammatical classes which were filled with arbitrarily-formed and combinable syllables to make words, e.g., Ruggles (1829) and Edmonds (1856), or took elements from existing languages (e.g., roots, prefixes, and suffixes). These elements were put together to create a new language, e.g., Esperanto (Zamenhof 1887), the best known of these creations.
Needless to say, it was the latter type, such as Volapük (Schleyer 1880), Interlingua (Peano 1903), Ido [1907] (de Beaufront 1919), Occidental (de Wahl 1922) and Interglossa (Hogben 1943) which were more successful (in a limited way) and easier to learn than the creations of Ruggles and Edmonds, but only if one was a European, as they all used the major languages of Europe as their basis. A speaker of an Algonquian or Bantu language would certainly have struggled to see the logic of such languages and would have found little to grasp in the system of word-formation. Nevertheless, such was the power and momentum of the movement towards artificial languages at the time, that, in 1903, Louis Couturat and Léopold Leau produced the massive Histoire de la langue universelle, an essential reference work on the subject, even today (Couturat & Leau 1903). (More recent broad studies of the artificial language phenomenon include those mentioned in my introduction to Haag’s work, above.)
Even at the time of Couturat and Leau, however, the idea of an international auxiliary language was changing. This is exemplified by the work of Carus (1904) whose ‘Suggestions for a Pasigraphy’ outlined a visual vocabulary and a syntax based on place-value, rather like a musical stave, an idea which went back to the work of Francis Lodwick published in 1666 (Salmon 1972).
The second set of influences on Haag was more contemporary and came not from the field of linguistics but from mathematics and logic.
Since the time of George Boole (b.1815), there had been a gradual trend towards bringing logic and mathematics together. In 1879, the German logician and mathematician Gottlob Frege (b. 1848) had published Begriffsschrift, eine der arithmetischen nachgebildete Formelsprache des reinen Denkens [Conceptual script, a formula language of pure thought modelled on that of arithmetic] (Frege 1879). Frege’s principal goal was to demonstrate that arithmetic could be reduced to logic. In the Leibnizian tradition, he recognised that to do so he would require an appropriate symbolism, just as arithmetical, geometrical, and chemical symbols allowed interpretation and calculation within their particular fields. The reduction of mathematical expressions and equations to logical propositions could be achieved by the use of letters for numbers and the introduction of symbols for arithmetical functions and logical operators (and, or, not, etc.).
He notes in his preface to Begriffsschrift, ‘If it is a task of philosophy to break the power of words over the human mind, by uncovering illusions that through the use of language often almost unavoidably arise concerning the relations of concepts, by freeing thought from the taint of ordinary linguistic means of expression, then my Begriffsschrift, further developed for these purposes, can become a useful tool for philosophers’ (pp.50-51). It should be noted that the Begriffsschrift is purely a pasigraphy, a language written to be read. There is no spoken version and it cannot be read aloud, other than by interpretation.
Not only did Frege reduce mathematical propositions to symbols, he also brought out logical structure through the use of an early type of tree-structure (similar to those later used by transformational grammarians) which showed how premises and conclusions were linked to together. Pure thought was thus given visual expression, but this did not represent a map of the mind. Such pure thought was expressed in the world through the script and truth functions could be calculated from the representation thus given.
As Legris (2012: 95) notes, ‘[I]t can be argued that the formal script was also conceived to represent formal structures. This representational side has […] the following main features: (i) there is only one structure (the world) to be directly represented, (ii) it is an ontological representation: it represents basic components of reality. As a result, the tension … between calculus and representation can be found also in the case of Frege’s script. This has been expressed traditionally by saying that Frege’s conceptual script is conceived as a universal language.’ Frege’s was not, of course, a description of natural language, but a limited and formalised system which went some way towards the ideal symbolic language of Leibniz.
Haag’s work, then, was carried out just at the time that universal language and logical representation occupied the thoughts of both intellectuals and idealists. The 1900 Paris conference on the possibility of an agreed International Auxiliary Language brought together both Esperantists, e.g., Couturat, and mathematical logicians, e.g., Russell and Peano. Haag’s little book of 1902 was yet to surface with its new ideas. Haag’s own work was in some ways influenced by both streams of thought but his own approach was to be both individual and innovative.
4. Haag’s Critique of Artificial Languages
Versuch opens with a damning critique of artificial languages of the Esperanto type:
Artificial languages such as the example discussed here are already in plentiful supply; they all have the practical aim of serving as a world language, and all of them remain just as far from this goal as from that of having any possible theoretical use, which is not [in any case] their aim. Without exception, they are based on existing language-types, but they simplify their inflectional systems. They draw their vocabulary haphazardly from existing languages. It is precisely because artificial languages randomly accumulate the plundered detritus which forms their core, that, from a logical point of view, they are fundamentally inferior to real and historically-attested languages; they often destroy both the logic and the aesthetic beauty of any inner conceptual interconnection and elaboration which, thanks to the root words, permeate a natural language when it is allowed to grow naturally, and they thus offend the mind as much as the sensitivities. (p.1) *
* All translations from Haag are by the present author.
Haag thus has no desire to create a language of the type described by Couturat & Leau as a posteriori. In the view of these two authors, what characterises a posteriori languages, such as Myrana (Stempfl 1889) or Universala (Heintzeler 1893), is the eclectic internationality of the vocabulary base and the grammar. It is this ‘plundered detritus’ which, in Haag’s view, is the principal weakness of the contemporary artificial languages, as logical structure is thereby destroyed. Such languages are thus neither a priori (created through an initial logical analysis of the world and bearing no resemblance to natural languages) nor mixed (created by combinations of elements drawn from natural languages, but insisting on invariable logical rules, much like a priori languages.).
Haag’s aim is different and its stands out in a peculiarly significant way:
Haag does not wish to create a new language which can be used, but to demonstrate the underlying logical structure of all language in a visual fashion.
We shall attempt to investigate how far we can express the conceptual content common to all human languages, without borrowing from any historically-attested language, thus making this conceptual content completely free-standing and independent. The expression plane must therefore abandon any traditional representation of sound, sacrifice the normal relationship between sound and symbol, and restrict itself to employing only mute symbols. In this way we shall arrive at a sort of logical picture-writing, a conceptual script into which the randomly evolving sense-based picture-writing of primitive cultures might well have developed, had it not become linked to sounds, an alliance in which it lost its independence. (p.2)
The presuppositions inherent in Haag’s belief in ‘conceptual content common to all languages’ and the possibility of its being ‘free-standing and independent’ will be discussed below in Section 14.
5. Terminology
Haag is concerned to show how language, and therefore thought, demonstrates its own logical structure. At the centre of his analysis lies the proposition or judgement (Urteil), the foundation stone of classical and medieval logic. From the time of Plato and Aristotle, logicians have concentrated on the truth or falsehood of statements and the logical means by which such values may be determined. Haag, however, shows little concern with such syllogistic demonstrations. His aim is to show that initial statements are tightly structured and to demonstrate how such structure may be shown in a symbolic, logical, but language-neutral way.
Haag’s basic analysis of a sentence is similar to the traditional grammatical analysis into subject (a person, thing, or topic about which something is said) and predicate (that which is said about the subject). In grammatical terms, of course, the predicate is everything in the sentence except the subject. Haag is more focused, however, and chooses a system which is more close to logic, that is, an argument-predicate structure, where argument is roughly equivalent to noun and predicate is roughly equivalent to verb or adjective. James Cooke Brown, the designer of Loglan (1975: 85) summarises such a structure: ‘Predicates make claims. Arguments designate the individuals, or sets of individuals, those claims are about.’ Thus, both The cat is large and The large cat show argument-predicate structure, although of different kinds. Both are equivalent to: There is some A such that, if A is a cat, then A is large.
This double form of predicate structure is reflected in Haag’s curious choice of vocabulary for his two logical sentence-elements, Träger and Verhalten. The former literally means carrier (from tragen, ‘to carry’ or ‘to bear’) and the latter, behaviour. These terms make both the understanding and an English translation of Haag’s text extremely difficult. The terms Argument and Prädikat already existed in logic in German but Haag chose not to use these. Nor did he choose Frege’s German terms Argument and Function [sic]. The author’s choice of terms which differ from these shows (a) that he was not beholden to any existing system of logic or contemporary approach to logico-mathematics, and (b) that he was trying to convey something slightly different. Indeed, there is no mention or acknowledgment in Versuch of the influence of any other writer, least of all Frege.
Haag’s notion of Träger corresponds in the main to that of the noun or noun phrase that fulfils the grammatical functions of Subject and Object (direct or indirect) in the sentence. A Träger is both a carrier of meaning (its referent) and of function, much like a tagmeme in the system of tagmemic analysis developed by Cook (1969) and Pike (1982). A tagmeme (unit) is both a grammatical slot and its filler, e.g., in The cat is large, The cat is a tagmeme having the reference [CAT] and filling the grammatical slot [SUBJECT]. In Haag’s system, the subject of the sentence is known as the unabhängiger Träger (independent Argument) and both direct and indirect objects are abhängige Träger (dependent Arguments).
Verhalten is more complicated. As noted, its central meaning is behaviour, but in Haag’s use the term covers both verbs and adjectives, i.e., it covers both action and attributes. It is also clear that Verhalten, in Haag’s idiosyncratic usage, bears a resemblance to Verhältnis, meaning relationship, i.e., the relationship of a verb or an adjective to a noun by virtue of an action depicted, or the attributes, qualities, or properties ascribed to the noun.
Haag is far more concerned with Verhalten than with Träger. Unlike the ‘language projectors’ of the 17th and 18th centuries (Wilkins, Dalgarno, de Maimieux, etc.), his aim is not to draw up a catalogue of things contained in the world, neatly classified into tables and columns, i.e., an a priori language. Rather, he aims to show in particular how mental concepts derived from bodily experience (e.g., near, far, above, below) can form the basis of a system of predication in which these concepts extend beyond their initial spatial field into the domains of mechanics, biology, and psychology. His neglect of Arguments, in particular, nouns, will be examined below in Section 14.
This concern with Verhalten reaches a degree of complexity when Haag takes a concept such as inside and notes that, depending on its context:
[It] produces the geometrical and geographical concept of entrance; in the anatomical field, pores, mouth, and so forth; from the mechanical point of view on the other hand, to penetrate, which is the essential characteristic of pointed objects, which, anatomically, gives us claws, thorns, etc.
Depending on its usage, then, a Verhalten can become a Träger (inside becomes to go inside, to penetrate, and ultimately the thing which goes inside, i.e., a claw or a thorn). Later semanticists such as Leech (1974) use the term predication downgrading for the process whereby a Verhalten (in Haag’s terminology) becomes a Träger, e.g., A person (Argument) who rides a bicycle (Predicate) becomes A bicycle-rider (Argument).
While no set of English logical terms corresponds exactly to Haag’s use, I shall here use the terms Argument and Function, following Frege’s usage, rather than that of the later Leech. This provides terminology which is contemporary with Haag and avoids any possible confusion between logical predicate (e.g., verb or adjective) and grammatical predicate (everything in a sentence which is not its subject). Furthermore, I shall retain the upper-case initial letter of the German to differentiate Haag’s use of Argument and Function from the everyday use of the words argument and function. It must, however, be borne in mind that Haag’s use of Function is very fluid, allowing changes in concept or category.
6. The Basis of Haag’s Conceptual System
Haag begins not by looking at language, but by looking at the human being and human perceptions. Language, he believes, derives from the fact that humans are embodied beings in a world which they perceive through their senses, and it is these senses and the perceptions which they provide that are the basis of thought and speech. The spatial is thus the central concept in Haag’s system. Concepts such as ‘time’ will later be derived from the central notion of ‘space’.
The four most important perceptions of space for the human being are the horizontal and the vertical, the distant and the enclosed, and relationships between objects are seen as obtaining in these fields. The first of these relationships are on the distance axis, namely near and far. Thus, A is near to B or A is far from B. From these may be derived in front of and behind, on the horizontal axis, orientation and visibility influencing the choice of term.
It should be noted here that Haag is primarily concerned with Functions, not with Arguments, i.e., he looks at the relationships of A to B, C to D, E to F, e.g., A is near to B, C is far from D and E is in front of F, etc. (as we have briefly seen in Section 5). However, these Functions can become Arguments, i.e., the symbols for proximity, distance, and precedence (nouns) will be based on the underlying spatial concepts and their symbols.
Haag expresses relationships such as the above symbolically:
The basic concepts of spatial Functions are indicated by means of dots and lines; the dot corresponds to the first Argument and the line to the second one. (p.39)
On the vertical axis come above and below: A is above B or A is below B.
Two other spatial perceptions are important (1) whether something is inside or outside another thing (2) whether something is open or closed. Haag thus arrives at the last four of his principal notions:
7. Force Levels
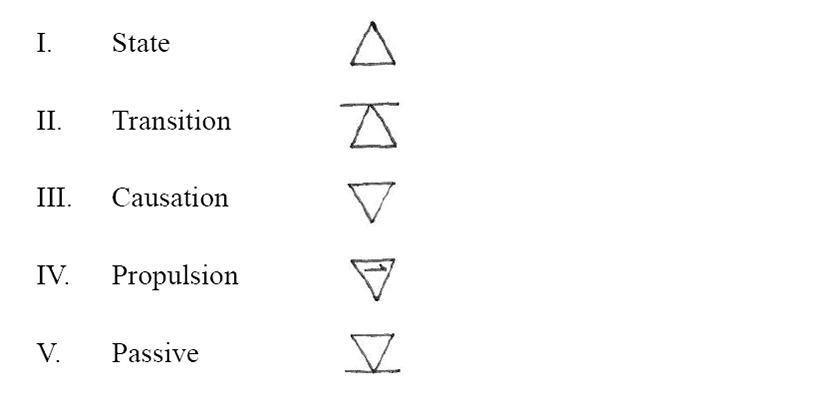
Before considering Haag’s other central concepts and their symbolic representations, it is necessary to introduce his notion of Dynamic Conceptual Levels or Force Levels, of which there are four, with a fifth expressing the passive sense.
Haag’s principal concepts (and many others) may be used at several levels, though not necessarily at all levels. They may described, using Haag’s Roman numerical notation, as follows:
Haag defines the differences between these Levels. Figures in square brackets have been inserted for clarity:
These concepts [II-V] may be understood as deviations from the concepts of ‘states’ [I] and they come into existence when we apply the most elementary concepts of time [II], type (or measure), [IV] and causality [III]. (p.6)
As an example, Haag writes:
The 7th spatial concept, inside, … produces at the state level (I): to be in, to occupy, to be enclosed; at the transition level (II): to go into, to occupy, to become enclosed; at the causation level (III) to insert, to cause to occupy (active) and to cause to be enclosed (passive) (V). As the first level expresses not only the state, but also the completed process, and the second the as yet incomplete process, one could regard them as opposites, perfect, and imperfect (but not in the temporal sense). (p.7)
Level IV (Propulsion) for inside would mean to force in, to drive in and Level V (Passive) would mean to be occupied.
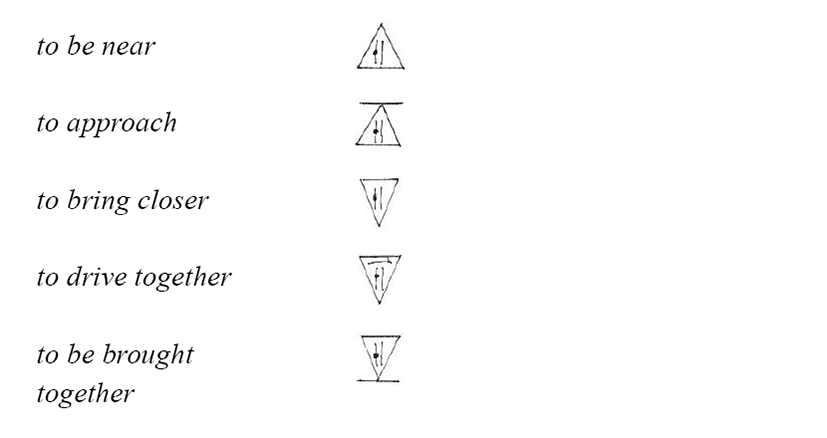
The first four of his concepts (near, far, in front of, and behind), used as Functions, would thus have the following meanings, according to the force level at which they were used:
To show at which Level a concept is being used, Haag uses triangular borders around its symbol. The levels are symbolised thus:
For the concept near, the combination of prime and border gives:
8. Additional Spatial Primes
On the basis of the first ten spatial primes (above), Haag creates a further ten which operate at Level II, i.e., Transition. Each is based on motion, relates to a source or goal, and corresponds to a prime at Level I (given in brackets in the following examples). Thus, at Level II:
(1)towards (based on near); (2)away from (based on far); (3)forwards (based in front of); (4)backwards (based on behind); (5)upwards (based on above); (6)downwards (based on below); (7)into (based on inside); (8)out of (based on outside); (9)closing (based on closed); (10)opening (based on open)
These are symbolized as follows.
A further six spatial primes relate to what might be described as a ‘transitory’ relationship with another person or thing. Haag notes:
Six spatial-dynamic concepts appear only on levels II and IV. They are not to be understood as forms of entry into the 10 states, which correspond to the spatial Functions of the Arguments; they are so to speak aimless and undirected movements. [My emphasis.] (p.8)
These six concepts are:
(11) along; (12) across; (13) (moving) over; (14) (moving) under; (15) back and forth; (16) through the middle
They are symbolised as follows.
9. Degree
The twenty-six primes (10 at Level I and 16 at Level II) provide the foundation-stone on which the remainder of Haag’s system is based. He later provides further symbols for more precise spatial concepts such as directly in front of, immediately below, etc., but we need not concern ourselves with these here.
More important than these additional space-related terms are those of Degree which can modify a term or express a relationship between two arguments involving measurement, negativity, or definition.
Closely related to spatial concepts are those of number and measure, and, in part, these derive directly from notions of touch, and, in part, from those of space. Much and little correspond to above and below, with allowance being made for the underlying concept of an intermediate quantity. The extreme limits appear as all and nothing.Near and far correspond to present and absent, all 6 of these being used in their literal sense. Similar and dissimilar [like and unlike] are directly related to near and far, in their relative senses. Unrelated to the above concepts are the remaining notions definite and indefinite, whole and partial (collective and individual in relation to many). (p.5)
Haag then justifies his use of mathematical symbols to represent these concepts (although he lists them in reverse order in his examples as shown below):
The general usefulness of mathematical symbols offers an additional reason to make these fundamental concepts the foundation of the system, despite their partial dependency on spatial concepts; taking this step is, however, justified because it means that the basic concept, especially in the latter [i.e., spatial] class can be extensively modified, which becomes essential to the creation of other concepts. It was (thus) possible to understand total and partial as a single concept. The same would, of course, be true for something and nothing (existence and non-existence) in relation to presence and absence; but the particularly appropriate applicability of the symbols ‘+’ and ‘–’ in the sense of expanding and limiting the above classes, means that these two are particularly suited to being independently related to the pure unmarked being, and that of ‘zero’ as equalling non-existence. In this way a further 11 symbols of degree have been added to the 10 spatial examples. (p.6)
Haag’s 11 symbols of degree, as shown in the original visual order are shown below in Table 2:
These concepts of Degree may be added to members of the spatial category and to others described below. Thus for the category of Space, the following emerge from the application of Degree:
10. Expanding the Categories: Fields and Senses
Haag now goes on to expand his basic cognitive notions into other fields, thereby expanding the possibilities both for predication (Functions) and entities in the world or the mind (Arguments). Rather than create arbitrary or even motivated symbols for each category, he chooses to use the initial letter of a Greek or Latin word which corresponds to his German nomenclature. Note: The original Latin or Greek has been deduced from Haag’s initials.
It is now possible to apply Haag’s concepts of Degree in these other fields. By way of example, the degree concepts 17-20, used in Table 3 in a spatial context, now produce the following meanings when used in other fields:
Note that for the most part these applications produce predications, i.e., Functions, but in the Typological application, an Argument may be produced, namely ‘feature’.
To the 26 spatial concepts and the 11 concepts of degree, Haag now adds 10 which derive from the senses:
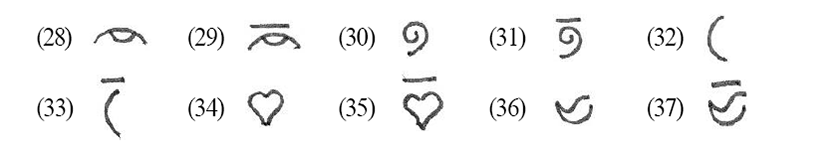
It becomes necessary here to add new concepts to the concepts of space and degree as foundations for the system and to extend this basis to the concepts of material reflectinglight, emitting sound, wet, warm, sweet and their opposites; that is to say 10 new numbers — 28 to 37 [Haag does not count the symbols of motion given at Level II in his drawing]. They play only a minor role in the system, in which they must appear almost entirely as a psychological class; they are not required in any others.
These are as follows:
(28) Light; (29) Dark; (30) Loud; (31) Quiet; (32) Wet; (33) Dry; (34) Warm; (35) Cold; (36) Sweet (and bitter); (37) Tasteless.
Symbolised as follows:
These concepts can now be applied in any of the 18 categories of Table 4, using the Force Levels and their appropriate symbolic borders (see p.75). The concepts loud and quiet, if placed at the Causation Level would mean to sound (tr.)/to silence; light and dark placed in this category would mean to illuminate/to darken. These concepts placed in the Feeling category would mean to hear/not to hear and to see/not to see. By way of further example, Haag notes:
In the thought category, the Causation Level itself [III] takes over as the means of expressing intention; to causeto be heard is to say; to cause to see is to show; to cause to know is to inform, such that this class is clearly divided into the two groups of knowing and informing, according to the Level that one uses.
Having established the 37 concepts and their symbols, Haag adds further symbolic combinations and variations, but their complexity and the lack of space within this paper do not permit me to deal with these.
11. Interlude
Thus far we have assembled the following elements which will go towards developing Haag’s ideas in greater detail:
-
1) A basic division between Arguments (essentially nouns) and Functions (essentially verbs and adjectives).
-
2) An initial list of 27 basic concepts, each based on bodily experience, motion, or degree and each symbolised in a particular way. The concepts of degree are based on concepts of space, e.g., more corresponds to above, and less to below, and, with the exception of ‘Non-existent’, the symbols of degree are organised in pairs of opposites (e.g., definite/indefinite).
-
3) Five Force Levels at which the concepts may operate: State, Transition, Causation, Propulsion, and Passive, numbered I-V. Thus the concept far would have the following meanings at different levels: I. to be away, to be absent. II. to go away, to remove oneself; to leave. III. to remove, to move away. IV. to send away (to drive away). V. to be removed/sent away.
-
4) A list of 18 fields in which the basic spatial concepts may operate in a more abstract and metaphorical way. The number of concepts is then increased to 37 through the addition of those which refer to the senses.
12. Deriving Syntactic Categories from Concepts
As outlined in Maun (2015), Haag is able to demonstrate that some concepts may be derived from others. Thus:
[T]he concept “to happen simultaneously” gives us, as a closer specification of a predicate, the preposition and conjunction “during/while” and the adverb “meanwhile”; the concept “to use” produces the preposition “for”, the concept “to exchange” produces “instead of”. (p.25)
Haag also demonstrates the converse, namely that adverbs can be transformed into verbs. Thus:
still = to continue; only (not until) = to follow; not yet = not begin; almost = to touch (in the favourable sense, positively); hardly = to touch (in the unfavourable sense, negatively); enough = to satisfy; only = separated, isolated.
These concepts can be used either as a more exact definition of the predicate, as a secondary predicate (adverb); or also as a primary predicate (verb) to the whole sentence as an argument (subject). ‘He’s still working’ or ‘His work continues’; both sentences consist of the 3 concepts: he, work, go on; the varying structure is expressed in the first sentence through the above-mentioned symbol for subordination; and in the second through the symbol for coordination. (p.25)
Before we examine the question of syntax, we must look at the way in which Haag derives words which will express the relationship of two ideas. These concepts are derived from the first concepts outlined in the system (e.g., proximity and distance). Setting out Haag’s rather dense paragraph on this subject in a more readable way than in the original, we find:
The adverbs ‘and, likewise, also, too, not only but also’ all come under the concept of ‘being present’;
the adverbs ‘only not, neither … nor’, the conjunctions ‘except that, without’ come under the concept of ‘being distant, missing’;
the adverbs ‘likewise, also, suchlike’ come under ‘agreement’ [i.e., equality];
the adverbs ‘but, on the contrary, but [on the contrary], however’ and the conjunctions ‘against which, whereas’ come under ‘disagreement’. They mean the same as the prepositions ‘with, without, like, unlike’;
for ‘or’, the classification ‘uncertain’ matches the concept of ‘exchange’.
In exactly the same way as we have treated logical relationships, for temporal ones we recognise the concepts ‘precede, follow, occur, coincide, follow immediately’ in which are concealed the prepositions ‘before, after, at the time of, in, within, immediately after’;
for causal relationships, ‘derive from, aim at, pass through, depend’, expressed through the prepositions ‘out of, to, through’;
for relationships of degree and manner: ‘be identical, be similar’, which correspond to the prepositions ‘like, unlike’. (pp.26-27)
13. Syntax
Armed with a vocabulary of Arguments and Functions and a selection of structural words derived from basic concepts, Haag is now able to address the question of syntax.
For the structural order of his propositions, he chooses a linear SVO order. The order of reading is left-to-right. Thus:
[T]he free argument, in which we seek the root of the Function, stands at the beginning; the dependent Argument, in which we seek the effect of the Function, is placed at the end. This construction forms the basis of each conceptual structure. By placing three concepts in this order, we arrive at a thought or expression of perception. The thought ‘Pleasure enhances life’ is entirely determined by three concepts: to take pleasure enhance live in this order, which expresses the causal relationship of the concepts. (p.22)
To represent things and phenomena (and things of unspecified nature) Haag uses the square as a symbol, and for people and animals he uses the circle. Thus the symbolic syntax for A is in B combines a square labelled A, the symbol for in (symbol 7 in his original list) surrounded by a triangle, indicating Level I (State) of this concept, and a further square labelled B. Thus:
To symbolise a transitive verb in use (C puts A into B), Haag places the subject first (a circle representing a person labelled C) followed by the concept in at level III (Causation) in an inverted triangle (see pp.69-70 for definitions of border use). The direct object A follows the verb and the adverbial phrase is represented by B. Thus
This proposition used at Level IV (C forces A into B) would thus be represented as:
Haag uses the following symbols for frequently-occurring words in his examples:
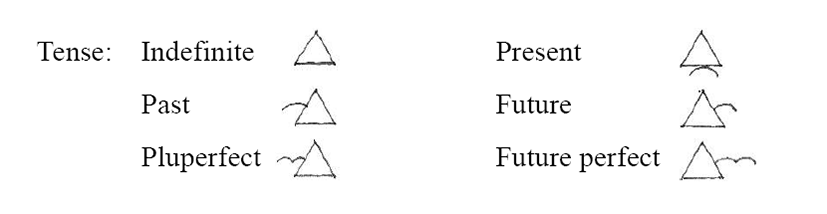
Tense markers are attached to the triangular borders of verbs. The orientation and form of the triangle will of course depend on the Force Level at which the verb is being used:
When written symbolically in full, a sentence such as He works so that he becomes rich requires a present tense marker, but as the time is actually unspecified, an abbreviated form with no tense marker is allowed, which conforms with Haag’s ‘Indefinite’ tense marker, i.e., an unadorned triangle.
Negation is expressed by a line over the element to be negated. However, the ‘zero’ symbol may be attached.
These are attached to Arguments. Haag recognises that a relative clause is, in fact, a full proposition which has been downgraded. Thus, The cat catches the mouse which is in the field is initially analysed as The catcatchesthe mouse [The mouse is in the field]. The relative clause itself is separated from the main clause by the use of square brackets. Within the relative clause, the anaphoric element (i.e., the relative pronoun) is represented by a vertical straight line. Thus, the above sentence becomes, symbolically:
Here the circles represent living beings and the square symbol a natural phenomenon (space). The Z represents the category ‘Zoological’, and N stands for ‘Nature’. The symbols > and < represent ‘large’ and ‘small’. These adjectives are not specified in the surface structure of the sentence in Haag’s prose text, but the symbols serve to clarify the relationship of cat and mouse. Later Haag shows how a relative clause such as The cat [which is large] can be further reduced to become The large cat.
Haag offers examples of adverbial clauses. Both main and subordinate clauses are normally placed within round brackets (as opposed to square ones used for relative clauses), but the brackets round the main clause may be dropped. In the example below, the symbol for behind at Force Level I is modified by the addition ‘T’ (time) to mean after. The sentence thus means I come after he writes.
Haag was certainly under no illusions as to the magnitude of his project, nor to the breadth and depth of his achievement, or rather, the lack of such breadth and depth. Versuch, he notes, was far from a perfected system. Indeed, it was only a foundation, which others could improve and on which they could successfully build:
Just as phonetic spelling made it possible to represent the endless variety of sound complexes of language as combinations of 20 to 30 letters, so it is possible to imagine a pasigraphy, which represents the vast range of combinable thoughts as combinations of a limited number of mental constructs. In addition to an alphabet of sounds, there must also be an alphabet of ideas. An attempt to create such a system has been made here, by breaking down the conceptual complexes of language into combinations of about 70 elements, which for the most part have been derived from each other. However, this is a crude language, and we admit that is the case, but nevertheless, from these 70 elements we can already create several thousand words.
We have to admit that the system created here is not only crude, but in many parts is extremely shaky, so that it often gives the impression of being a house of cards; but on the other hand it does have some notably stable parts borrowed from established logical practice, which convince us that the whole undertaking was not some illusion, and that even if the weaker parts were to collapse, something capable of development would remain. So let it be left to others who are better skilled in logic than I to correct and sharpen the basic outlines, to complete and extend the content, and to surround with a much firmer frame those parts which may yet give way. (p.49)
It is now possible to examine the truth of Haag’s modest claims and to demonstrate how linguists in modern times have unconsciously reflected his ideas in ways which show promise for future development in fields such as ‘Real Character’.
14. Critique of Haag: A Man of His Time
In critiquing Haag’s system, it must be borne in mind, firstly, that it is only a Versuch, an outline, a mere 83 pages of ideas, and, as Haag stresses, it is theoretical, not practical. Wilkins (1668) thought that his own 300-page Essay was insufficient to define a universal language, but there is a difference between Wilkins and Haag. George Dalgarno criticised Wilkins for failing to reduce his system to absolute primes. Haag, however, has successfully done this, giving a sound outline which may only require some extension or reorganisation.
On the other hand, Haag, was bound by history and by the ideas prevalent at the time (1902). He did not have the benefit of the further century of practical and theoretical work on languages and linguistics which we enjoy today. We shall therefore examine the negative aspects of his work with a certain degree of tolerance before going on to examine the positive side and his important contribution to symbolic language, including a modern Real Character.
On the other hand, Haag, was bound by history and by the ideas prevalent at the time (1902). He did not have the benefit of the further century of practical and theoretical work on languages and linguistics which we enjoy today. We shall therefore examine the negative aspects of his work with a certain degree of tolerance before going on to examine the positive side and his important contribution to symbolic language, including a modern Real Character.
Unlike many previous writers, Haag does not begin his attempt to create a symbolic language by analysing the world into categories. Where the ‘language projectors’ of the 17th century and later writers produced extensive lists of words (mainly nouns), Haag prefers to leave such categorisation alone while he concentrates on semantic primes and ‘logical’ structure. As we have seen above, his use of ‘fields’, to which Verhalten may be applied at various levels, provides a limited range of tangible concepts, e.g., ‘inside’ can give the noun ‘claw’ in the Mechanical field, and in the field of Space can give the noun ‘door’. However, when it comes to symbolising concepts such as ‘cat’ or ‘mouse’, the system goes no lower than the level of ‘biological entity’, and Haag is obliged to write the name of the animal below the symbol. This is clearly a shortcoming, as a nominal designation in written form must appear in some natural language, not in symbolic form. The same is true of most verbs. ‘Wrote’ is symbolised only as a ‘cultural act’ with the German word schrieb written below it.
In his introduction Haag states: ‘We shall see how far the conceptual content common to all human languages can be expressed’. Haag makes the basic assumption here that conceptual content is common to all human languages. While we shall find much to commend his analysis of perceptual and semantic primes, it is evident that languages use these primes in different ways.
Having picked out the vertical and horizontal perceptual axes as the basis for his system, Haag now has a ‘currency’ of units such as ‘near’, ‘far’, ‘in’, ‘on’, etc. However, even Standard European languages do not use these units in exactly the same way. In English, a key may be said to be inthe door. In French, it is sur la porte (on the door). In German, no preposition is used. Instead the verb stecken is used and no mention of place is required: Der Schlüssel steckt (roughly, The key projects). Batchelor & Offord (1982) devote no less than 26 pages of their book to differences between French and English usage of prepositions, and Pinker (2007) points out that while most perceptual distinctions are binary (e.g., here v. there), the distinction is relative, not absolute, and some languages such as Spanish make a three-way distinction between ‘near me’, ‘far from me’, and ‘in between’. Tlingit, a language spoken in the Yukon, has a four-way distinction, adding ‘very far from me’. Haag’s alleged primes are thus very far from being ‘conceptual content common to all human languages’.
In the field of syntax, functional order certainly isn’t universal. Haag’s SVO order within the proposition is very European. A Japanese user of Haag’s system would be obliged to read first the Subject, then go to the end of the sentence to read the Object and then go to the middle to find the Verb, since Japanese is an SOV language. As Haag was a speaker of Turkish, he would have known that that language also uses an SOV order.
Speakers of Celtic languages (Welsh, Scots Gaelic, Erse, Breton) would have to start with the Verb, since these languages use the VSO order. Very few languages use an OSV order, but if Haag’s system were to be ‘universal’, then it should have been capable of accommodating such languages. Clearly it isn’t, at least, in its present form.
Even symbolic languages do not necessarily use an Argument-Function arrangement, corresponding to Haag’s SVO order. In symbolic logic, Functions precede Arguments. Thus A is x is expressed as x [A]. Haag’s syntactic system thus flies in the face of both natural and symbolic languages. His description of his system as being built auf logischer Grundlage (‘on a logical basis’) is not strictly true. Its structure, working from perceptual and semantic primes to the symbolic representation of propositions is, at best, systematic, not logical.
Similarly Haag’s tense system (indefinite [i.e., generic present] – present – past – pluperfect – future – future perfect) is not based on logic, but corresponds neatly to that of Standard European languages, e.g., French or German, but is inadequate for the representation of more ‘exotic’ languages, i.e., those outside the well-known European languages of the time. Deutscher (2010) cites the Matses language, spoken along the Jivari river, a tributary of the Amazon, by about 2,500 speakers. In this language the temporal foci of tenses are widely different from those of European languages. Thus past tenses are chosen depending on whether the event is seen in as being in the recent past (up to a month ago), in the distant past, (roughly from a month to fifty years ago) or remote past (more than fifty years ago).
This is not to say that Haag’s system is incapable of making such distinctions of tense and evidentiality, just as European natural languages can do so. Deutscher (2010) notes that the Matses distinctions can be handled in English by such circumlocutory expressions as ‘I saw with my own eyes a short time ago that …’, ‘I inferred a long time ago that …’, ‘I guessed a very long time ago’, and so on.
On the other hand, where Haag’s system (in the form given) has insufficient means to express such meanings through the verb, in some cases it forces the writer (this is a pasigraphy, remember) to give information which for writers of some languages would not be necessary. Thus Haag requires that tense be indicated on the symbol for the verb, but there are languages in which tense is not indicated, e.g., Mandarin, Vietnamese, and Samoan (Crystal 1987).
Thus Haag’s statement ‘We shall see how far the conceptual content common to all human languages can be expressed’ is both too ambitious and theoretically unsound, since it assumes that there is ‘conceptual content common to all languages’. This in part derives from the tenets of the time at which he was writing and his own studies. The historical relationships of languages (particularly of the Indo-European group) showed that similar grammatical categories seemed to be assignable (sometimes incorrectly), much on the models of the Latin grammars of Donatus and Priscian, and the influence of the Port-Royal grammarians with their aim of revealing ‘the unity of grammar underlying the separate grammars of different languages’ (Robins 1967) was long-lasting.
At the time (1902), of course, serious data-driven research into languages other than those of the Indo-European group was in its infancy (Powell’s classification of Amerindian languages was only published in 1891), and the possibility that languages could exhibit non-European concepts and syntax and non-European grammatical categories had barely dawned on linguists. The era of descriptive as opposed to historical linguistics was yet to come.
Since Haag’s era, the concept of ‘universals’ has experienced both good and bad times. In the era of Sapir and Whorf the general belief was that languages could vary infinitely, i.e., there were no universals, and that the language that one spoke influenced the way that one saw the world, e.g., Sapir (1921) and Whorf (1941). The 1960s saw research into a wider field of languages which provided evidence of commonality, e.g., formal universals (e.g., patterns of word order) and substantive universals (e.g., syntactic or phonological categories) (Greenberg 1963). This was considered to be evidence for what Chomsky calls Universal Grammar. More recently, however, Evans & Levinson (2009) have shown that there are very few alleged universals which appear in all languages, and that the few recurring patterns which are found are better seen as ‘stable engineering solutions satisfying multiple design constraints, reflecting cultural-historical factors and the constraints of human cognition.’ Deutscher (2010) gives further support to the notion that some supposedly universal perceptions, such as colour, are, in fact, partly constrained by culture and language.
Haag’s attempt, therefore, to find ‘the conceptual content common to all human languages’ was doomed to failure from the start, but he was in part a victim of historical ideas and in part a man born before his time. Only later would ideas appear which reflected Haag’s thinking. Until now, he has never received acknowledgement, because of the obscurity of his work, which (a) was published in a booklet, not in an accessible academic journal, and (b) was written in German, rather than in French or English, the academic languages of the time.
15. Haag Renewed: Modern Linguistics
It seems that, in his lifetime, Haag’s ideas were virtually unknown in Europe and the United States, as his name does not figure in bibliographies or surveys. Nevertheless, he reached many conclusions about the nature of language and its ways of working long before respected linguists (and philosophers) whose work currently enjoys favour. He was clearly a deep-thinking and far-sighted linguist whose ideas outstripped the thinkers of his time. His was a quiet revolution.
It would be both unfair and inaccurate to suggest that Haag actually influenced future researchers but his prescience in a number of semantic and syntactic areas is quite remarkable. These include the fields of embodiment, metaphor, and force levels.
Lakoff & Johnson (1999) believe that it is the fact of having bodies and perceptions that is the starting point for our ability to use language:
Spatial relations concepts are at the heart of our conceptual system. They are what makes sense of space for us. They characterize what spatial form is and define spatial inference. But they do not exist as entities in the external world. We do not see spatial relationships the way we see physical objects. (p.30)
This is, by chance, virtually identical to Haag’s starting point:
The perception of ‘space’ forms the basis for the organisation of our concepts. Spatial perceptions must therefore serve as the elements which we use to express our thoughts in a logically constructed manner. Because these perceptions are intimately linked to vision, they provide the most direct means of making thought accessible to the senses. (1902: 2)
Haag was thinking along these lines at the beginning of the 20th century, long before Lakoff & Johnson, and reached exactly the same conclusions.
Furthermore, Haag’s system anticipated another aspect of Lakoff & Johnson’s work, that of metaphor. I have drawn attention to Haag’s method of representing concepts in different fields through the use of his basic bodily concepts (or semantic primes, to put it another way). Thus in front of as a spatial concept, can become precedence as a temporal concept, or, to convert an Argument into a Function, to precede, to come before. Similarly, non-existent becomes never when applied temporally and to be unreal when applied logically (see above, Table 5).
This, of course, is to use the basic concepts in a metaphorical manner, although Haag does not use the term ‘metaphor’, preferring ‘transference of meaning’. Lakoff & Johnson note:
Metaphor allows conventional mental imagery from sensorimotor domains to be used for domains of subjective experience. For example, we may form an image of something going by us or over our heads (sensorimotor experience) when we fail to understand (subjective experience). […] Conceptual metaphor is pervasive in both thought and language. (1999: 45)
We are not concerned here with literary metaphors, e.g., Shakespeare’s ‘It is the east and Juliet is the sun’, but rather with ‘understanding and experiencing one kind of thing in terms of another’ (Lakoff & Johnson 1980: 5). For these writers, metaphor is not an add-on to language; it is an essential part of the system and the way in which we shape language to our everyday needs. ‘Primarily, on the basis of linguistic evidence, we have found that most of our ordinary conceptual system is metaphorical in nature’ (1980: 4).
Using the prime metaphors of Grady (1977), Lakoff & Johnson list a large number of examples which, when examined, reveal themselves to coincide with many of Haag’s prime concepts.
Haag, of course, works the other way. To convey happy, he would take a concept such as up and move it into his category of Sensation. He also has the advantage of being able to place his primary symbols at a given force level, thereby quintupling his possibilities for metaphors.
Whichever way one works, be it from a concept to a semantic prime (Lakoff & Johnson) or from a semantic prime towards a concept in a particular field (Haag), one is working with metaphor. Lakoff & Johnson have developed their ideas more thoroughly and more systematically over the years, but Haag’s insights into the way in which semantic categories work through metaphor preceded theirs by some 80 years. This is certainly testament to Haag’s intellectual ability and his foresight.
Another aspect of language that has come to the fore in recent years is that of force. We have discussed Haag’s notions of ‘force levels’ in some detail above (Section 7). Again he was far in advance of his time. It was not until the 1970s and 1980s that linguists such as Len Talmy (e.g., Talmy 1976) began to examine semantic distinctions in which the notion of ‘force’ separated one concept from another. Thus the notion of ‘fall’ is distinct from ‘fell (a tree)’ as force and causation are involved in the latter, c.f. Haag’s Levels II and III.
Pinker (2007) deals with force in verbs, particularly with those constructions which can take two forms, depending on the focus of the verb (or Function, in Haag’s terms) on its surrounding constituents (Arguments, for Haag). Thus John smeared grease on the axle can also be formulated as John smeared the axle with grease, the first of which is used if the axle is seen as Location, the second as Patient. Verbs such as brush, daub, and plaster work like this in English, while other verbs resist this usage. Those such as ladle and insert cannot be used in this way — He ladled syrup into the jar v. *He ladled the jar with syrup or He inserted cotton wool into his ear v. * He inserted his ear with cotton wool.
Pinker points out that a notion which is common to some of these verbs, but which crosses the two types, is that of force. Verbs such as brush, daub, splotch, spatter, stuff, cram, and inject all involve a degree of force, ‘With the brush verbs, the agent applies force simultaneously to the stuff and the surface […]. A jointly felt force is also present in the stuff verbs, where the contents and the container are pressed up against each other […]’ (2007: 53). Haag’s system covers the notion of applying force, but would require some expansion to cope with the double-construction verbs and the forces, surfaces and movements involved.
While Haag does not deal in any detail with the influence of semantics, and thereby force, on syntax, Croft & Cruse (2004) take up the notion of ‘the force-dynamic structure of events’ (c.f. Haag’s terminology — ‘Dynamic Conceptual Levels or Force Levels’) as being a determinant in the encoding of syntactic constructions, e.g., ‘I baked Brownies for Mary’ v. ‘I beat the eggs with a fork’. The choice of ‘for’ in the first sentence is necessitated by the fact that ‘Mary’ is the endpoint, the beneficiary of the baking event, while ‘with’ is necessitated in the second sentence, as ‘the fork acts upon the eggs and is therefore an intermediate participant in the force-dynamic chain’ (Croft & Cruse 2004: 66). Ideas first developed by Haag have thus been independently expanded and developed by linguists and philosophers of today. One can only wonder what progress would have been made if these ideas had been noticed in 1902.
While Haag does not deal in any detail with the influence of semantics, and thereby force, on syntax, Croft & Cruse (2004) take up the notion of ‘the force-dynamic structure of events’ (c.f. Haag’s terminology — ‘Dynamic Conceptual Levels or Force Levels’) as being a determinant in the encoding of syntactic constructions, e.g., ‘I baked Brownies for Mary’ v. ‘I beat the eggs with a fork’. The choice of ‘for’ in the first sentence is necessitated by the fact that ‘Mary’ is the endpoint, the beneficiary of the baking event, while ‘with’ is necessitated in the second sentence, as ‘the fork acts upon the eggs and is therefore an intermediate participant in the force-dynamic chain’ (Croft & Cruse 2004: 66). Ideas first developed by Haag have thus been independently expanded and developed by linguists and philosophers of today. One can only wonder what progress would have been made if these ideas had been noticed in 1902.
Haag’s aim was to produce a graphic representation of the logic underlying language. Many of today’s linguists have been content to demonstrate semantics in terms of logical representation and syntax in terms of tree diagrams and transformations. More forward-thinking researchers have chosen to follow a route which was pioneered by Haag, even if his proposals never came to public attention.
Thus, Chilton (2014) develops what he calls Deictic Space Theory and uses many of the ideas which first appear in Haag, though without ever (apparently) having come in contact with his work. In words which echo Haag’s introduction to Versuch, he states ‘What I am suggesting is that there is a derived conceptual foundation that uses spatial cognition of various kinds, and that language in turn uses it as the most fundamental requirement for communication.’ (p.4). Thus, ‘To be situated means, for human language users, that our use of language will always assume and/or refer to the place and time of speaking, and will take a perspective on the surrounding physical environment.’
Chilton’s theory is, quite naturally, more developed than Haag’s and he summarises it as follows: ‘The most general hypothesis is that linguistically prompted conceptual representations are consistent with, and in evolution exapted [sic] from, more basic cognitive functions — in particular, visual attention, frames of reference, locomotion and action schemas, all of which arise from an organism’s interaction with physical space’ (pp.299-300).
For Chilton, both objects and ideas are situated in space, the former in physical space, the latter in mental space. The speaker stands at the centre of the world of discourse and his/her meanings will be defined by the physical and mental space which surrounds him/her. With ideas, the ‘space’ is metaphorical, i.e., there is an analogy between physical distance and mental proximity or distance. Thus, ‘this’, a mental designation, refers to something close to the speaker either physically (this book) or mentally (this philosophy) and ‘that’ refers to something which is either physically or mentally more distant (that knife v. that dogma).
By adopting the mathematical notion of a vector, Chilton is able to combine the notion of direction and magnitude. A vector consists of a straight line in the form of an arrow, with its tail originating at the speaker and its head some distance further away. Concepts are placed along the line in a linear but not in a scalar way, i.e., distance is relational, not literal. Thus, on the distance vector, ‘that’ occurs on the mental vector of ‘Distance’ and is ‘further away’ mentally from the speaker than ‘this’. This corresponds with Haag’s symbolic representations (symbols 1 and 2), in which the focus-dot is close to the line for ‘here’ or ‘this’, and further away from it for ‘there’ or ‘that’. Haag uses empty space where Chilton uses a vector arrow.
Chilton also posits a temporal vector and a modal one. The temporal vector represents the ‘distance’ in time from the speaker. Thus, ‘now’ is at the point of origin, ‘yesterday’ at a short distance and ‘two years ago’ much further along the line. Note how this corresponds closely to Haag’s transfer of notions of distance into the temporal (T) category. Haag’s temporal dislocations for the spatial concepts of ‘in front of’ and ‘behind’ thus produce the verbal forms:
-
1. to precede/predate, before a given time; a) to be past/over, before the present; b) to be early, before the usual time.
-
2. to succeed, after a given time; to be in the future, after the present; b) to be late, after the usual time. – II. b) to recur (p.53).
[The ‘b’ reference is to duration, as opposed to all the others which are punctual, i.e., non-durational.]
The third vector, the modal vector, is a semantic space which runs from realis to irrealis, that is to say, from that which is real, actual, known, and certain to that which is unreal, indefinite, unknown, and uncertain. Thus, I is at the starting point (tail) of the realis vector; he is further along and fairy is at the far end (head). Similarly, factual sentences couched in the indicative mood (Paris is the capital of France) would be at the realis end of the modal vector, whereas counterfactual sentences are much further along the vector, nearer the head (If I’m ever rich enough, I might possibly to move to Utopia).
Remarkable as it may seem, Haag covered such cases in Versuch. Under the concept of Cause, he notes:
The prepositions out of, through, and to correspond to the conjunctions, because, if, with the result that, in order that; if these are negated, we get although, even if.
He become rich out of (he work) means ‘He becomes rich because he works.’ — The sentence ‘He would become rich if he worked’ is differentiated from the above example not because of the relationship between individual propositions but by their lack of definition. We must therefore ensure that, in the ‘because’ clause, the definiteness and factuality of the individual propositions are expressed; the symbol for time serves this purpose, in this case the present. If the time symbol is omitted, the relationship becomes uncertain or hypothetical. ‘His actual wealth is derived from his actual work’, as opposed to ‘His possible wealth is derived from his possible work’.
The hypothetical value of the relationship, where the time symbol is missing, would in principle apply throughout, but, for the sake of simplicity, we need only insert the time symbol when there is the possibility of misunderstanding, as here; if, on the other hand, we want to express uncertainty clearly, the periphrasis with be possible is available to us (at its simplest as an attribute before the bracket); — by putting the symbol for past next to the preposition: His possible wealth was the result of his possible work, we put the conditional clause in the past: He would have become rich if he had worked. […]
The effect of denying effect and consequence is to obtain concession: He works not that (he become rich) means in surface form: His work does not lead to his becoming wealthy, or; Although he works, he does not become rich; or, in conditional form: Even if he worked, he would not become rich. (pp.30-31)
By using simple prepositional concepts (out of, through, to) Haag is able to deal with counterfactuals in a different way from Chilton, but in a way which is based on his own theory and which covers the data to be explained. Again, Haag, writing over a hundred years before Chilton, is both prescient and perspicacious.
16. Haag and a Modern ‘Real Character’
The notion of a modern digital ‘Real Character’ has been discussed in Maun (2013, 2015). A ‘Real Character’ (hereafter, RC) is a pasigraphy, a symbolic language meant only to be read, not pronounced. The type of system outlined in the two papers mentioned above involves icons, indices, and symbols, with syntactic function being indicated by position (place-value). Such a system has no fixed word order and the glyphs (characters) can be read in the order natural to a native speaker of any given language. For further details, see the two papers in question.
The form that an RC might take is yet to be determined in detail. Maun (2015) suggests that icons, in the Peircean sense, should be used for concrete concepts (e.g., man, dog, butterfly) and that a degree of conventionalisation will be required. Thus humans may be pictured face-on, animals, side-on, and insects from above. This aspect of the development of an RC presents few difficulties.
The use of indices, on the other hand, might be more difficult. Smoke is an indicator of the presence of fire, and clouds an indicator of forthcoming rain. To use pictures of smoke and clouds, however, would be ambiguous. Is the reference to smoke or to fire? Do the clouds represent themselves or rain which is about to fall? Such ambiguities would not be desirable in a system which is designed to convey meaning instantly through visual form and the use of indices would therefore be rare in a developed RC.
The representation of abstract concepts is certainly the most difficult task for the development of an RC. Abstractions cannot, by their nature, be pictured directly, and it therefore becomes necessary to develop a symbolic system by which such meanings may be conveyed. Now, it is often stated in the literature on symbols and symbolic languages, that symbols, in the Peircean sense, are arbitrary and bear no resemblance to that which they represent, e.g., the symbols for a switch or a resistor in an electric circuit are entirely conventionalised and look nothing like switches or resistors.
It is not necessarily the case, however, that such symbols need be entirely arbitrary and Haag’s system offers a basic vocabulary of symbols which may be used both in their primary sense and which may also provide the basis for the metaphorical uses which Haag demonstrates in his section on transference of meaning (see Table 6).
Thus, in a sentence such as The office is above the shop, the basic symbol No. 5 (above) will be used. Haag also points out that metaphorical extension leads to the following type of example:
In the category of emotion it is necessary to ensure the inclusion of the concept which is brought about by the use in the literal sense, as well as by that of Level III, e.g., in the case of the spatial concept in its relative sense. I: A is above B, translates as A looks down on/despises B —
[…] ‘A is high’ is interpreted as: ‘A is arrogant’ — Level III (literally): ‘C raises A’, interpreted as ‘C respects A’ (p.12).
Thus any developed symbol which represents looks down on/despises, arrogant, or respects will incorporate the basic symbol for above (symbol 5) with some extension to indicate metaphorical use in a social category.
Haag’s system of symbols thus offers a basic vocabulary for both literal and metaphorical use. This is not to say that all problems of abstraction are solved by such use. RC is meant to readable by any reader, whatever his or her language. If, for instance, there were a language in which ‘respect’ was conveyed by the notion of ‘looking at someone’s feet’, then the notion of ‘respecting’ would have to be conveyed by a symbol indicating that the respecter is above the respected, and not vice versa.
Such problems are bound to occur, since a metaphor in one language is not necessarily translated in the same spatio-temporal terms into another, viz. the examples of keys in doors, above. However, close analysis of concepts might reveal commonality at a more basic level, e.g., whether the ‘respecter’ is above or below the ‘respected’, the basic concept involved is not locational. Rather, the concept of ‘inequality’ is involved, or, in more basic terms, ‘not the same’. It thus becomes possible to use Haag’s symbols of Degree, rather than of Space. The resolution of such issues remains a problem for the future.
Haag’s basic concepts and the symbols that represent them go along way to providing a lexicon of symbols which may combined in a Leibnizian manner to demonstrate meaning which may be either real or metaphorical. The use of colour has been recommended in Maun (2013) to distinguish metaphorical from literal meaning.
Haag’s standard European left-to-right layout of a sentence assumes the syntactical ordering of Subject-Verb-Complement (or Object). Since this is not the ordering for many languages outside the European model, it will be necessary to alter the positions in the sentence at which functional units may appear. Thus the T-bar arrangement suggested in Maun (2013) might be adopted.
In this arrangement, the Verb appears above the T-bar, with Subject and Complement (or Object) in the two angled spaces below. The sentence may then be read in the order of the reader’s native language, e.g., Japanese, S-C-V. Some languages, e.g., Welsh and Irish will be read V-S-C and certain more unusual languages will use the order V-C-S, e.g., Malagasy, Tzotzil. Even the rare C-V-S order, e.g., Hixkaryana and Apalai, and C-S-V, e.g., Jamamadi, Apurina are thus catered for (examples from Crystal 1987). (It should be noted that we are concerned here with actual surface structure, not any ‘underlying’, theoretical SVC deep structure which may be assigned to all languages as in Chomsky’s Minimalist Theory. See Seuren 2004 .)
Such a syntactic device even allows for dialectal variation. In pronominal use in Standard English, the indirect object must precede the direct object — Thus, I gave the box to the boy becomes I gave him it. In Northern dialects in England, however, the reverse order is quite normal — I gave it him. If the indirect object is placed below the vertical bar of the T, this allows both alternative versions as the reader can read direct and indirect objects in the order which is most linguistically natural, i.e., I gave him it or I gave it him. Note, too, that the two versions of the nominal sentence are also possible in this representation — I gave the box to the boy or I gave the boy the box.
The T-bar arrangement thus allows Haag’s symbolic vocabulary, together with RC icons, to become readable in a way that his 1902 language is not. The European syntactic basis gives way to an adaptable and flexible system with a greater pan-linguistic basis. Since a Real Character is intended to be read by all, this solution offers a definite syntactic improvement on that suggested by Haag.
Basic symbols founded on sensorimotor perception are not the only way in which Haag’s ‘graphical language’ may be incorporated into a modern digital RC. His notion of ‘force levels’ re-discovered by Lakoff & Johnson, Pinker, Croft & Cruse, and, more recently, Chilton, inter alia, is also a powerful addition to the means of such expression.
Haag’s idea of using a triangular border around a symbol to indicate its force level might be adopted, but if the symbols became multifarious or complex, then the triangle would become ever larger in order to contain them. This could lead to complications in presenting them. The following is offered as a solution for each level, with these diacritics preceding a Haag symbol (or symbols):
-
Level I: - (representing a base-line, stasis, absence of force)
-
Level II: : (representing 2)
-
Level III: [ (representing ‘C’ for causality)
-
Level IV: :: (representing 4)
Level V (passive) could thus be represented by the dice-face symbol for ‘5’. This style of representation is simple and matches Haag’s use of lines and points for his symbols.
An as-yet unresolved problem is that of representing the type of verbs described by inker (2007) such as brush, daub, and inject, which involve force, motion and direction. This is not as difficult as might at first be thought. A verb such as brush involves the concepts of ‘on (a surface)’ and ‘movement back and forth’. These have already been provided by Haag, in the form of Symbols 4 (‘above’) and 15 (‘back and forth’), and they could be combined at Level III, using the ‘[’ symbol. Daub, which is more vigorous in its meaning than brush, might involve the same symbols, presented as being at level IV. Inject could combine Symbol 7 (‘in’) with a ‘[’ symbol representing Level III, together with Symbol 32 (‘wet’), since injection necessarily involves a liquid.
The question of Location and Patient dealt with by Pinker (2007) (see above, Section 15.2) is also relatively easy to deal with. Any sentence in symbolic form is presented using T-bar syntax (see above, Section 16.2). Since Location and Patient in Pinker’s sentences are questions of emphasis (e.g., He loaded logs onto the lorry v. He loaded the lorry with logs), the emphasised element may simply be marked by underlining. The reader then reads the T-bar sentence in an order appropriate to his or her language for the understanding of an emphasised element.
17. Conclusions
Karl Haag’s little book Versucheiner graphischen Sprache auf logischer Grundlage contains a wealth of insight which pre-dates modern research and has unfortunately been ignored, forgotten, or neglected. His aim was not to create a new artificial, symbolic language, but to demonstrate the logical basis on which a pasigraphy might be created, such that meaning would be deducible from form.
Haag deserves credit and his insightful system calls for closer examination and development, particularly by those whose interest lies in artificial languages, universal linguistic systems, and Real Character. Haag’s name does not appear in any of the major surveys of constructed languages. The time has come for him to be included in any further major work resembling those of Couturat & Leau (1903) or Okrent (2009).
Perhaps Haag deserves an epitaph that reflects his concepts and his relevance to the researchers of today. I would suggest:
Haag’s work is not yet over.